
To prove Newton's second Law
Using Fletcher's Trolley

Thomas O’Sullivan’s Leaving Cert. Maths & Physics Notes.
Experiment: To show that the acceleration of a body is proportional to the applied force and inversely proportional to the mass of the body.
Apparatus: As in diagram:
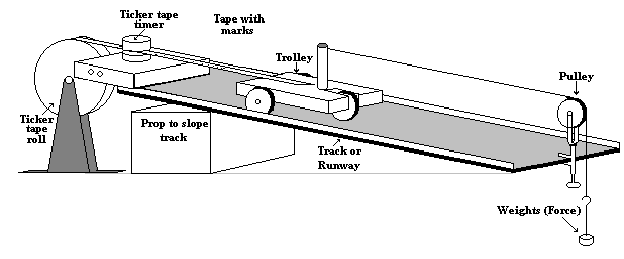
Theory:
Newton's Second law states that "the rate of change of momentum of a body is proportional to the force causing it and takes place in the direction of that force".
i.e. (mv - mu) / t µ F or m(v - u) / t µ F which gives ma µ F.
Outline of experimental procedure:
This experiment is divided into two parts.
Experiment A: To show that a µ F.
4. As the trolley moves, the ticker tape, which is attached to it, passes through the ticker tape timer and the resultant dots can be used to find the acceleration.

To calculate the acceleration:
5. Repeat steps 3 and 4 for forces of 0.2N, 0.3N, etc..
Results:
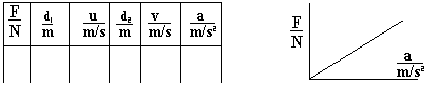
Conclusion: A straight-line graph shows that F is directly proportional to a. (the slope of this graph actually gives us the mass of the trolley.)
Experiment B. The second part of this experiment is to show that a µ1 / m.
Results:
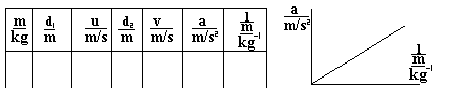
Conclusion: A straight-line graph shows that a is inversely proportional to the mass. (The slope of this graph gives us the weight in the scale pan (0.5N above)).
From parts A and B of this experiment we can deduce that F µ ma.
Note:
Tilting track to overcome friction
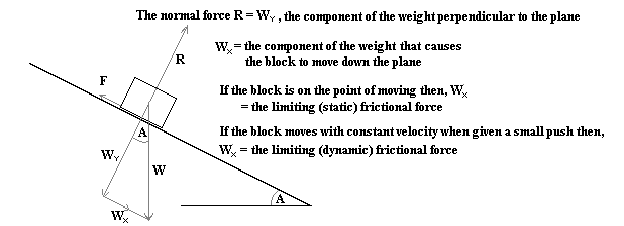
If the track is tilted then a component of the body's weight, Wx acts down the slope in the opposite direction to F, the frictional force. If the trolley, when given a slight push moves down the slope with constant velocity, then the overall force down the plane is zero (no acceleration, Newton's first Law) and F (Limiting (dynamic) frictional force) = Wx.
From the diagram above:
![]()
giving Wy = W Cos A
![]()
giving Wx = W Sin A
then
![]()